CONTENIDO
- 1. Mifflin-St Jeor
- 2. PSU (2003b)
- 3. Brandi
- 4. Mifflin-St Jeor x 1.25
- 5. Faisey
- 6. Modified Penn State PSU (2010)
- 7. Penn State 2003a Invalidated by EAL in 2007 and 2009
- 8. Swinamer
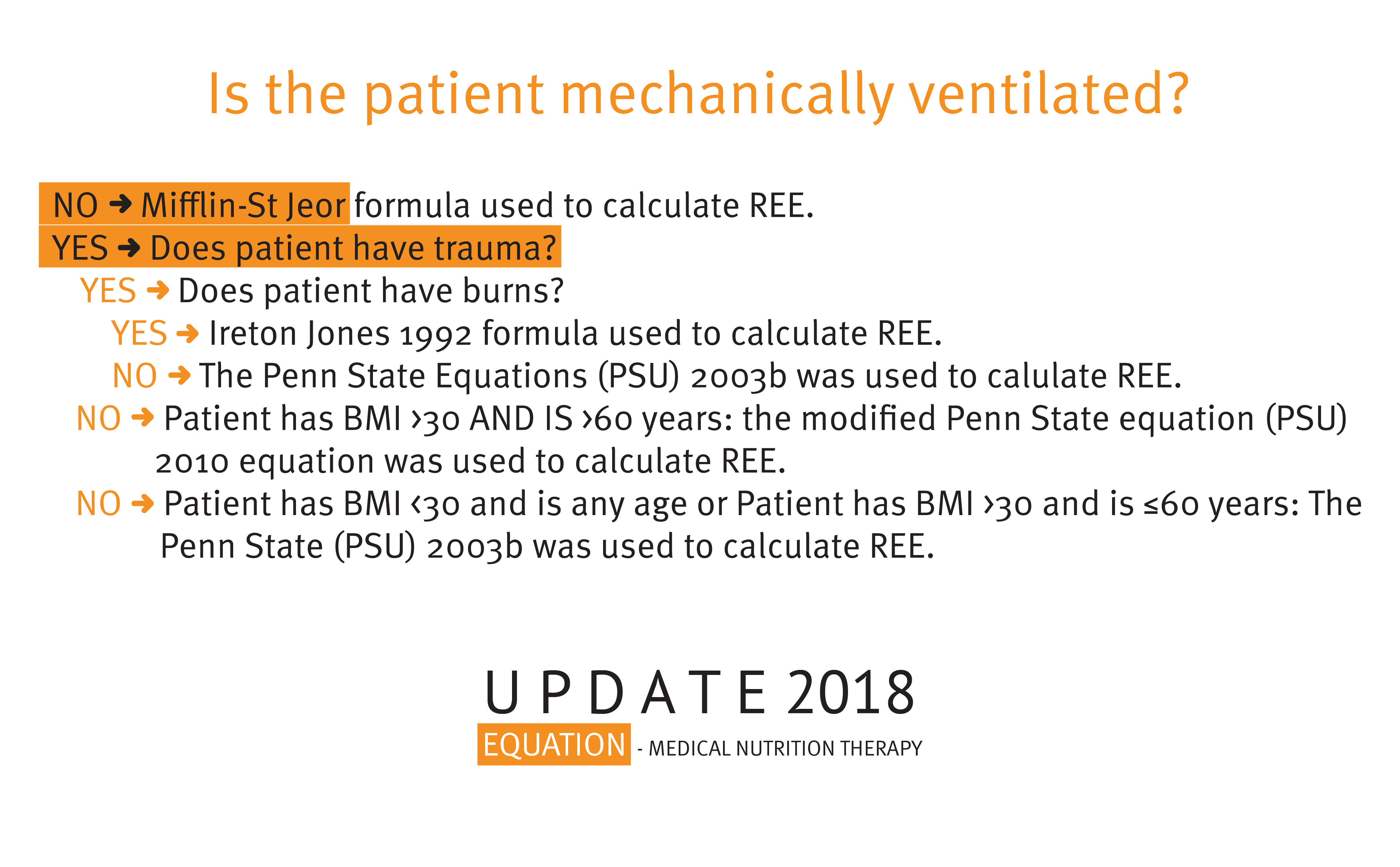
- 9. Ireton-Jones 1992 11. Penn State 1998
- 10. Penn State 1998
- 11. Harris Benedict
- 12. Owen
- 13. WHO/FAO/UNU
- 14. Dietary Reference Intakes (DRI)
- 15. Kcal/kg EAL in 2007 and 2009
CONDICIONES
- Adult Weight Management — Ambulatory
- Chronic Kidney Disease (CKD) + Post Kidney Transplant (after surgical recovery)
- Chronic Obstructive Pulmonary Disease (COPD)
- Critical Illness — Non-obese
- Critical Illness — Obese Patients
- Critical Illness —Obese Patients, Aged 60 years and Older
- Critical Illness — Non-obese
- Critical Illness — Mechanically Ventilated, Obese
- Disorders of Lipid Metabolism
- Gestational Diabetes — Non-obese - Obese
- Heart Failure
- Human Immunodeficiency Virus (HIV)/Acquired Immune Deficiency Syndrome (AIDS)
- Oncology - Breast Cancer - Head and Neck Cancer—Chemoradiation Hematologic Malignancies—Hematopoietic Stem Cell Transplant (HCT)
- Spinal Cord Injury Acute Phase
- Spinal Cord Injury Rehabilitation
- Unintended Weight Loss in Older Adults - Weight maintenance —healthy older adults
- Unintended Weight Loss in Older Adults - Weight Maintenance —Underweight Older Adult
Actividades solo para asistentes con donativo
1. Realiza la pre evaluación antes de visualizar el vídeo:
2. Realiza la evaluación final posterior a visualizar el vídeo:
3. La constancia digital llegará a su correo electrónico (junto con el material) y la física a su dirección postal.
Actividades para asistentes en general
1. Realiza la pre evaluación antes de visualizar el vídeo:
MÁS INFORMACIÓN
REQUISITOS
Ser estudiante, pasante o egresado de carreras o posgrados de Ciencias de la Salud, principalmente, relacionados con la Nutriología Médica.
Limitado a 1,000 estudiantes. Fecha limite de registro 15 de julio de 2018.
AVAL ACADÉMICO
16 horas curriculares / 1 crédito académico Colegio Mexicano de Licenciados en Nutrición de Jalisco A.C. Dirección General de Profesiones - Secretaría General de Gobierno Registro SEP 73/C112-1
CONSTANCIA
¿No realizaste donativo?, realiza un donativo de $1,500.00 y recibe la constancia a tu domicilio y el material del curso a tu correo.
PLATAFORMA LIBROS
¿Te interesa un acceso anual a la Plataforma de Libros?, la anualidad cuesta 3,000.00, sin embargo, para los que se registraron al curso con o sin donativo a solo a $1,500.00
INVERSIÓN
El curso es gratuito patrocinado por el Instituto Mexicano de Nutriología Clínica A.C. y el Colegio Mexicano de Licenciados en Nutrición de Jalisco A.C.
DURACIÓN
El estudiante cuenta con un periodo para completar el curso, del 20 al 21 de julio de 2018.
BECAS ECONÓMICAS DIPLOMADOS
Proceso:
- 1. Seleccione un diplomado (25 disponibles) visite la página o solicité lista de diplomados al +52 1 33 1833 3115 (solo WhatsApp).
- 2. Solicite la beca y consulte disponibilidad en el diplomado que seleccionó al +52 1 33 1833 3115 (solo WhatsApp).
- 3. Si fue autorizada su beca. El periodo para realizar el Pago de Beca para Diplomado es en los 7 días posteriores, favor de solicitar beca solo, si está 100% seguro que la va a aprovechar. En caso de no completar el pago durante el periodo, la beca pasa a otro interesado y no podrá solicitar otra beca. Precio normal del diplomado es de
$34,000.00+ $2,500.00, el precio con beca es de 8,000.00 del diplomado + $2,500.00 de Trámite Administrativo (se cubre 15 días previos al inicio). - 4. Seleccione un calendario de inicio: octubre 2018 (duración 6 meses), enero 2019, abril 2019, julio 2019, octubre 2019.
Equations
A table with a list by condition, formula name and equation, guideline, and evidence is available following these equations.
Equations
A table with a list by condition, formula name and equation, guideline, and evidence is available following these equations.
Brandi Equation
HBE(0.96) + HR(7) + VE(48) – 702
HBE = Harris-Benedict Equation; VE = Expired minute ventilation
Faisey Equation
W(8) + H(14) + VE (32) + T(94) – 4,834
Equation uses weight (W) in kilograms and height (H) in centimeters. VE is expired minute ventilation and T is body temperature in degrees centigrade.
Harris-Benedict Equations
Men: Resting metabolic rate (RMR) = 66.47 + 13.75 (W) + 5 (H) – 6.76 (A)
Women: RMR = 655.09 + 9.56 (W) + 1.84 (H) – 4.67 (A)
Equation uses weight (W) in kilograms (kg), height (H) in centimeters (cm), and age (A) in years.
Ireton-Jones Energy Equations (IJEE) 1992
Spontaneously breathing IJEE
(s) = 629 – 11(A) + 25(W) - 609(O)
Ventilator dependent IJEE
(v) = 1925 – 10(A) + 5(W) + 281(S) + 292(T) + 851(B)
Equations use age (A) in years, body weight (W) in kilograms, sex (S, male = 1, female = 0), diagnosis of trauma (T, present = 1, absent = 0), diagnosis of burn (B, present = 1, absent = 0), obesity more than 30% above initial body weight from 1,959 Metropolitan Life Insurance tables or body mass index (BMI) more than 27 kg/m2 (present = 1, absent = 0).
Mifflin-St Jeor
Men: RMR = (9.99 X weight) + (6.25 X height) – (4.92 X age) + 5
Women: RMR = (9.99 X weight) + (6.25 X height) – (4.92 X age) – 161
Equations use weight in kilograms and height in centimeters.
Owen
Men: RMR = 879 + 10.2 X weight
Women: RMR = 795 + 7.18 X weight
Penn State Equations (PSU)
Also known as PSU 2010 (Modified Penn State Equation)
RMR = Mifflin(0.71) + VE (64) + Tmax(85) – 3085
Used for patients with BMI over 30 and older than 60 years old. Validated in 2010 by the ADA Evidence Analysis Library (EAL).
PSU 2003b (Penn State Equation)
RMR = Mifflin(0.96) + VE (31) + Tmax (167) – 6212
Used for patient of any age with BMI below 30 or patients who are younger than 60 years with BMI over 30. This equation was validated in 2009 by the EAL and is also referred to as the Penn State Equation.
PSU 2003a (Penn State 2003a)
Invalidated in 2007 and 2009 by EAL
RMR = HBE(0.85) + VE(33) + Tmax (175) – 6433
[use actual weight in all patients]
Swinamer Equation
EE = 945(BSA) – 6.4(A) + 108(T) + 24.2(BPM) + 81.7 (VT) – 4,349
Equation uses body surface area (BSA) in squared meters, age (A), temperature (T) in degrees Celsius, breaths per minute (BPM), and tidal volume (VT) in liters per minute.
World Health Organization (WHO)/Food and Agriculture Organization of the United Nations (FAO)/United Nations University (UNU)
Weight only
Men
Women
Weight and height (m)
Men
Women
Table
Brandi
Mifflin-St Jeor x 1.25
Faisey
PSU (2010)
Invalidated bye EAL in 2007 and 2009
Swinamer
Ireton-Jones 1992
Penn State 1998
Non-obese
Obese
~70% of DRI
Breast Cancer
Head and Neck Cancer—Chemoradiation
May be higher during acute graft-versus-host disease (GVHD) and/or for patients receiving >75% of total daily energy from parenteral nutrition
Injury factor of 1.2 and an activity factor of 1.1
27.9 kcal per kg for those with paraplegia
Weight maintenance —healthy older adults
Weight Maintenance —Underweight Older Adults
Higher for weight gain
How is body composition related to resting metabolic rate?
The mass of tissue that performs metabolic functions determines the resting metabolic rate. The basic unit of metabolism is the cell. Therefore the metabolically active mass is the body cell mass. However, the fat-free mass is typically mentioned as the active mass. The difference between the fat-free mass and the body cell mass is that fat-free mass includes the body cell mass but also its support structure, which has no metabolic activity. The fat-free mass contains a wide variety of tissues with substantially different metabolic rates (Elia, 1992). Thus the brain, liver, kidneys, and heart, which make up 7% of the weight of “standard man” account for 84% of the resting metabolic rate, while muscle mass, which constitutes 40% of body weight, makes up 16% of the resting metabolic rate (Elia, 1992; Muller, 2002). Although there are big differences in the resting metabolic rates of the tissues that constitute the fat-free mass, there is a strong correlation between total fat-free mass and resting metabolic rate. There is also a correlation between fat-free mass and body weight such that body weight correlates with resting metabolic rate. This correlation is strengthened by the addition of sex (men usually have more fat-free mass than women), age (older people usually have less fat-free mass than younger people), and height (for a given weight, a taller person will generally have more fat-free mass). Thus body weight, height, age, and sex correlate with resting metabolic rate through a collinear relationship with fat-free mass and body cell mass (Mifflin, 1990), but it is the body cell mass that determines resting metabolic rate.
How is resting metabolic rate best estimated in acutely ill patients?
Equations exist for calculating resting metabolic rate specifically for acutely ill patients (ie, hospitalized patients who are not mechanically ventilated in a critical care unit) (Ireton-Jones, 1992). However, no validation studies exist, so it is unknown if these equations are proper to apply. There does exist limited data testing the validity of healthy predictive equations (Harris-Benedict and Mifflin-St Jeor) in acute care patients. In one of these studies the unmodified Harris-Benedict equation was validated against measured resting metabolic rate and found to predict accurately 47% of the time (Gariballa, 2006). These investigators found a correlation between resting metabolic rate and C-reactive protein, but the strength of correlation was low (R2 0.21 after adjusting for body size). In another study the resting metabolic rate of hospitalized acute care patients and chronic care clinic patients was measured (Weijs, 2008). Several pertinent findings resulted from this study. On average the resting metabolic rate was increased about 10% in acute care and about 5% in clinic patients using the unmodified Mifflin-St Jeor equation as the base equation. However, the variability was wide, ranging from 30% above the Mifflin value to 40% below it. Unmodified Mifflin-St Jeor values predicted the resting metabolic rate of acute care patients only about 50% of the time.
Lacking tested population-specific equations for acute care, and recognizing that healthy calculation standards generally do not predict very well the resting metabolic rate of acutely ill patients, the two most common methods of estimating the metabolic rate are to calculate one of the equations for healthy people and then multiply the result by a stress and/or activity factor, or by calculating the metabolic rate in kilocalories per kilogram of body weight. Neither method is validated.
Stress factors are usually based on disease type, and they are mean values of ratios between measured resting metabolic rate and predicted healthy resting metabolic rate. There are several problems with this method. First, it is very likely that increased resting metabolic rate is not a function of the type of illness but of the inflammatory response to it. Second, the factor is a mean of a range of measured values, so it will match only a small portion of patients to which it is applied (only those whose true expenditure is near the mean of the published data). Third, the predicted healthy resting metabolic rate calculation is most often the Harris-Benedict equation, which is being replaced in clinical practice by the Mifflin-St Jeor equation. Mifflin-St Jeor values tend to run about 5% lower than Harris-Benedict values. Fourth, across a range of body sizes from underweight to morbidly obese, the actual ratio between measured metabolic rate in the ill and predicted healthy resting metabolic rate changes, resulting in a larger stress factor in small people and a smaller stress factor in larger people.
How is resting metabolic rate best estimated in critically ill patients?
The Penn State equation appears to offer the most accurate prediction of resting metabolic rate in critically ill mechanically ventilated patients. The accuracy rate of the equations is about 75% if accuracy is defined as a prediction that is +10% or less of the true resting metabolic rate (Frankenfield, 2009). This equation has been validated in a total of about 500 patients. It has been shown to be accurate over time (Frankenfield, 2012). In fact, daily computation of the resting metabolic rate with the Penn State equation over 7 days is as accurate as a single measurement of resting metabolic rate that is extrapolated over the same 7 days. Metabolic rate must be measured twice per week to be more accurate than daily computation with the Penn State equation. The equation works well in obese people, even morbidly obese people, but not as well in underweight patients, in whom it is accurate only about 50% of the time (Frankenfield, 2012).
How is resting metabolic rate best estimated in healthy people?
The relationships between resting metabolic rate, body cell mass (fat-free mass), and weight, height, age, and sex are utilized to estimate energy expenditure in healthy people (Mifflin, 1990). Most of these estimation methods are linear regression equations that use some combination of weight, height, age, and sex. These equations were based on samples of nonobese and obese people, drawing a single line through the data and therefore intended for use in both obese and nonobese people. The Mifflin-St Jeor equation appears to be the most accurate of these linear equations (Frankenfield, 2003). However, the lines relating body weight to resting metabolic rate are not exactly the same in these populations, so this approach has the potential for creating error in one or the other. A few equations are nonlinear, using a power function to express the allometric relationship between body weight and resting metabolic rate (Livingston, 2005). This should matter most in underweight or severely obese people. However, when such equations are tested in validation studies, they do not improve the accuracy rate over that for the linear equations (Livingston, 2005).
Should hypocaloric feeding be practiced in the critically ill patient?
In the 2009 A.S.P.E.N. guideline, hypocaloric feeding in the first week of critical care was recommended. The Academy of Nutrition and Dietetics critical care work group concurred with this recommendation. There is evidence that relative hypocaloric intake, especially for obese patients, may be of benefit early in the course of the critical illness. Therefore, the nutrition prescription for energy may be 60% to 70% of the total energy needs determined during nutrition assessment. However, this does not mean automatically that the nutrition support regimen should be calculated to deliver energy at the 60% to 70% range. If the patient is fed enterally (ND-2.1), the tube feeding product and rate should be calculated to meet 100% of the energy requirement, and then the registered dietitian should monitor to assure that, with all the feeding interruptions that occur during a critical care stay, the actual energy delivered is in the 60% to 70% range (see monitoring and evaluation above). For critically ill patients receiving parenteral nutrition (ND-2.2), achievement of the 60% to 70% targeted intake should be calculated directly into the parenteral nutrition admixture because parenteral nutrition is not interrupted as is enteral nutrition.
The nature of the guideline writing process means that the data supporting these recommendations likely pre-date 2008. Since these recommendations were published, two important studies have emerged that could cause reconsideration of the hypocaloric feeding concept (Singer, 2011; Heidegger, 2011). In both of these studies, achievement of energy balance improved clinical outcomes. Importantly, both of these studies were prospective randomized trials, whereas most previous research on this topic was based on retrospective or prospective observational data. There are four other important points regarding one of these studies (Singer, 2011). The first is that the energy gap was not allowed to persist for long, yet it was found to be detrimental. Subjects who had not reached more than 60% of energy balance via enteral feeding by critical care Day 3 were randomized to start supplemental parenteral nutrition or to continue with enteral nutrition alone. The second is that the difference between hypocaloric feeding and energy balance groups was fairly small but very much in line with the level of underfeeding recommended by A.S.P.E.N. The final energy balance in the hypocaloric feeding group was 73 ± 27% of energy expenditure. The third is that energy balance was measured rather than assumed or estimated (expenditure being measured with indirect calorimetry). The fourth is that the energy gap was closed by using supplemental parenteral nutrition, meaning that the positive effect of energy balance on outcome was strong enough to overcome the presumed negative effect associated with the use of parenteral nutrition.
What are the components of total energy expenditure? What is the relative contribution of each component to total energy expenditure?
Total energy expenditure is composed of basal metabolic rate as well as the thermogenic effects of food and physical activity (Volp, 2011). Basal metabolic rate is fairly constant compared to the other two components. All three components can be affected by chronic, acute, and critical illness.
Basal metabolic rate is the largest component of total energy expenditure. It is defined as the level of energy required by the body to support vital functions (organ activity, maintenance of cell membrane potentials) and occurs when an individual is in a fasting state and is at absolute rest, awake but immediately following sleep. Energy expended in the basal state is difficult to measure because it requires a person to sleep overnight at a testing center or for the testing to occur in the person’s home. Therefore, resting metabolic rate measurement is often used as a substitute for basal metabolic rate. Resting and basal metabolic rate are nearly the same thing. The principal difference is that resting metabolic rate allows for some physical movement between awakening and testing, followed by a period of recovery before measurement (Frankenfield, 2009). For healthy individuals, basal or resting metabolic rate represents 50% to 75% of total energy expenditure.
The amount of energy utilized for the mechanical and chemical breakdown, absorption, and storage of nutrients is referred to as the thermic effect of food. It persists for up to 5 hours after a meal and can raise the metabolic rate by 7% to 9% of energy consumed (Compher, 2006).
Energy expenditure related to physical activity is highly variable and should be carefully considered when estimating total energy expenditure. Physical activity energy expenditure can be divided into exercise and non-exercise activity thermogenesis (NCM). Non-exercise activity thermogenesis includes activities of daily living and working, fidgeting, and maintaining posture. Exercise activity thermogenesis is energy expended during purposeful exercise. Exercise activity thermogenesis can account for 15% to 30% of daily total metabolic rate, and non-exercise activity thermogenesis can account for 15% to 50% of total daily metabolic rate. However, it is unusual in industrialized societies for the two components of physical activity energy expenditure combined to approach 50% of the total daily metabolic rate.
The effect of illness on total energy expenditure is balanced between a tendency toward increased resting metabolic rate and a tendency toward lower expenditure on physical activity. Illness that produces an inflammatory response raises the resting metabolic rate (Frankenfield, 1997; Gariballa, 2006). This increase can be anywhere from 0% to 75% of healthy resting metabolic rate. Energy expenditure on physical activity can be just as variable. Some acutely ill patients ambulate and some do not or cannot. Physical therapy encompasses activities from range of motion to strength and gait training, and energy expended on such activities is similarly variable. Whether an acutely ill patient who undergoes physical therapy compensates for this activity by sleeping more afterward has not been studied, but such an effect has been shown in the elderly (Govan, 1992). In critical care, some nursing activities can raise the metabolic rate by nearly 30%, but these are episodic and of short duration, so the overall effect on total metabolic rate is closer to 5% (Weissman, 1984).
Thermogenic effect of feeding occurs in the ill person who eats a meal. However in circumstances where an individual receives continuous infusion of nutrition support, the thermogenic effect of feeding is negligible (Frankenfield, 2012; Heymsfield, 1987).
What body weight is the proper one to use in resting metabolic rate equations?
When calculating resting metabolic rate in overweight or obese people, actual body weight should be used in most metabolic rate equations because actual body weight was used in the development of these equations. Use of adjusted body weight will result in underestimation of true resting metabolic rate in almost every case (Frankenfield, 2012a). Likewise in underweight people, no adjustments to the actual body weight should be made because these will cause resting metabolic rate to be overestimated. However, if resting metabolic rate is being calculated as kilocalories per kilogram (kcal/kg), then the value may be underestimated by as much as 25% if actual body weight is used (Hoffer, 2003).
What is the application of energy assessment within the Nutrition Care Process using International Dietetics and Nutrition Terminology?
Determination of metabolic rate is fundamental to the nutrition care of the patient, and it impacts each step of the Nutrition Care Process. A number of pieces of information need to be assembled in the nutrition assessment step. Based on the discussion above, these data include frame size (AD-1.1.3), age (CH-1.1.1), and gender (CH-1.1.2) and are used to calculate predicted healthy resting metabolic rate, which serves as a reference standard and also as a component of many other calculation methods. Data that might help in determining if hypermetabolism is present include vital signs (PD-1.1.9) such as body temperature, heart rate, and respiratory rate (depending on whether the patient is on a ventilator or breathing on his or her own); minute ventilation; leukocyte count; and the patient’s medical history and current diagnoses and conditions (CH-2.1). Measured resting metabolic rate (BD-1.8.1) or calculated resting metabolic rate is used to determine the patient’s total estimated energy needs (CS-1.1.1), recorded in the nutrition assessment section under the heading of Comparative Standards. The method used in determining needs (CS-1.1.2) should be stated (eg, healthy predicted resting metabolic rate x stress factor, illness-specific equation x activity factor, measured resting metabolic rate x activity factor).
The measured or calculated resting metabolic rate can be used to diagnose an increase in energy expenditure (NI-1.1), by comparing this value to the reference standard of Mifflin-St Jeor with no modifiers for illness or activity and using actual body weight for all patients, including the obese and underweight. It is known that as a reference standard the Mifflin-St Jeor equation will sometimes be in error (about 20% of the time). It also must be remembered that just as there is a range of normal values in most reference standards, a range of +10% is typically allowed in metabolic rate reference standards. Therefore a nutrition diagnosis of increased or decreased nutrient needs (energy) should not be made unless the calculated or measured resting metabolic rate is more than 10% different from the calculated Mifflin-St Jeor value. Other nutrition diagnoses that may be appropriate include:
Nutrition intervention includes the determination of goals, a nutrition prescription, and nutrition strategies to meet goals and provide the nutrition prescription. For patients in whom energy balance is the goal, the nutrition prescription for energy will match the total estimated energy needs calculated in the Comparative Standard of the nutrition assessment step of the Nutrition Care Process. For patients who have a goal of weight gain, the prescription for energy will be higher than the value calculated in the Comparative Standard, and for patients who have a goal of weight loss the energy prescription will be lower than the Comparative Standard.
Actual energy intake (FH-1.1.1) is an important nutrition care indicator to follow in the monitoring and evaluation step of the Nutrition Care Process. Actual intake should be compared to the energy prescription, which in turn will be a factor of the total estimated needs determined in the Comparative Standard. If the actual intake does not match the prescription, the feeding plan should be adjusted (increased or decreased) to promote actual intake that meets the nutrition prescription.
What modifiers if any are needed for total energy expenditure estimates in critical care?
Modifiers (multiplication factors) for physical activity are probably necessary for critical care metabolic rate equations and for measurements of resting metabolic rate. For sedated patients this multiplier is not more than 10% and more likely is about 5% (Weissman, 1984; Frankenfield, 1994). For nonsedated patients the modifier is difficult to quantify except to say that the more motion the higher the factor. There is little evidence to guide the selection of a modifier in this situation. One study in critically ill children noted a mean increase in total energy expenditure over resting of 22% (Van der Kuip, 2007). However, this mean value obscures a wide variability in the contribution of physical activity to the total energy expenditure in critical care. In this study of 20 patients, 6 had total energy expenditure that was 1.44 to 2.0 x measured resting metabolic rate, while 8 had total energy expenditure that was equal to resting, and the remaining 6 had total energy expenditure that was less than 1.15 x the measured resting rate. Four of the patients were not sedated or mechanically ventilated during the study, but it is not know whether these 4 patients were in the group with large differences between total and resting metabolic rate. An analysis of the degree of sedation and movement should be included in the energy assessment of the critically ill patient.
Multiplication factors for stress level are not required for measured resting metabolic rate measurements or for any equation that was developed from critically ill patients (Penn State, Faisy, Swinamer, Ireton-Jones). However, if a healthy standard such as the Mifflin-St Jeor or Harris-Benedict equation is used then a stress factor is required. This approach is not recommended because the existing multiplication factors are based on means and therefore will only be accurate in a fraction of patients.
A multiplier for thermogenic effect of feeding is probably not necessary in critically ill patients receiving continuous feeding infusion (Heymsfield, 1987). First, thermogenic effect is minimized or eliminated in patients being fed by continuous infusion (assuming no overfeeding) (Heymsfield, 1987). Second, most critical care predictive equations were developed from data on fed patients, so thermogenic effect if it exists is contained in the equation (alternatively, in patients whose metabolic rate is measured, the measurement protocols usually stipulate that the feeding not be interrupted for the measurement so any thermogenic effect of feeding is captured in the measurement).
Why and how is resting metabolic rate measured?
Measurement of metabolic rate is more accurate than estimation. Methodology is oriented toward resting rather than total energy expenditure measurement (Compher, 2006). The reason for this is fourfold: (1) resting metabolic rate is the most reproducible of the components of total metabolic rate; (2) it is the largest component of total energy expenditure; (3) it is comparable to a reference standard; and (4) it is the most feasible component to measure. Reproducibility and comparability are important for the purpose of nutrition diagnosis. Measurement of resting metabolic rate is more feasible than measurement of total energy expenditure because it can be accomplished in as little as 15 minutes whereas for total energy expenditure, measurements must be extended for several hours or must be repeated multiple times per day.
Indirect calorimetry is used to measure metabolic rate. To accomplish a resting metabolic rate measurement, attention must be paid to room conditions and patient state (Compher, 2006). Measurements should be conducted in a thermoneutral environment. If possible the room should be quiet and dimly lit (Compher, 2006). Ventilator settings should be stable for at least 30 minutes before a test is performed. Continuous feedings do not need to be stopped for the measurement (Compher, 2006; Frankenfield, 2012). There can be no air leaks and the fraction of inspired oxygen should not exceed 60%. The calorimetry device should be warmed up and calibrated per manufacturer specifications. Minimal patient movement or stimulation should occur during the measurement. The measurement should last 10 to 30 minutes (determined by protocol) and the coefficient of variation of the measured oxygen consumption and carbon dioxide production should be within established limits for the time length of the measurement. The first 5 minutes of the measurement should be discarded (Compher, 2006), although this might not be necessary in heavily sedated patients (however, if not discarding these data, they should be compared to the subsequent data for consistency).
Although resting metabolic rate is the largest portion of the total energy expenditure, the two are not always equivalent. Multiplication factors can be used to approximate total metabolic rate from a resting measurement.
REE/EEE Calculator Equations
Ireton-Jones (IJEE) 1992 EquationVentilator dependent IJEE (v) = 1925 – 10(A) + 5(W) + 281(S) + 292(T) + 851(B)
Equations use age (A) in years, body weight (W) in kilograms, sex (S, male = 1, female = 0), diagnosis of trauma (T, present = 1, absent = 0), diagnosis of burn (B, present = 1, absent = 0)
Men: RMR = (9.99 X weight) + (6.25 X height) – (4.92 X age) + 5 Women: RMR = (9.99 X weight) + (6.25 X height) – (4.92 X age) – 161 Equations use weight in kilograms and height in centimeters.
Penn State Equations (PSU)PSU 2010 (Modified Penn State Equation)
Used for patients with body mass index (BMI) over 30 and aged more than 60 years old. This equation was validated in 2010 by the American Dietetic Association Evidence Analysis Library (EAL); it is also referred to as the Modified Penn State Equation. RMR = Mifflin(0.71) + VE (64) + Tmax(85) – 3085 [with Mifflin formula inserted: RMR = (W(10) + H(6.25) – A(5) + 166MALE –161) 0.71 + Tmax(85) + MINUTE VENTILATION(64) – 3085]
PSU 2003b (Penn State Equation)Used for patient of any age with BMI below 30 or patients aged younger with 60 years and a BMI above 30. This equation was validated in 2009 by the ADA EAL; it is also referred to as Penn State Equation. RMR = Mifflin(0.96) + VE (31) + Tmax (167) – 6212 [with Mifflin equation inserted: RMR = (WT(10) + HT(6.25) – AGE(5) + 166MALE -161)0.96 + + TMAX(167) + MINUTE VENTILATION(31) -6212]
REE/Estimated Energy Expenditure Calculator Results Diagram
Referencias
- Compher C, Frankenfield D, Keim N, Roth-Yousey L; Evidence Analysis Working Group. Best practice methods to apply to measurement of resting metabolic rate in adults: a systematic review. J Am Diet Assoc. 2006;106(6):881-903.
- Elia M. Organ and tissue contributions to metabolic rate. In: Kinney JM, Tucker HN, eds. Energy Metabolism: Tissue Determinants and Cellular Corollaries. New York, NY: Raven Press; 1992:61-77.
- Frankenfield DC, Ashcraft CM. Description and prediction of resting metabolic rate after stroke and traumatic brain injury. Nutrition. 2012;28(9):906-911.
- Frankenfield DC, Ashcraft CM, Galvan DA. Longitudinal prediction of metabolic rate in critically ill patients. JPEN J Parenter Enteral Nutr. 2012;36(6):700-712.
- Frankenfield DC, Ashcraft CM, Galvan DA. Prediction of resting metabolic rate in critically ill patients at the extremes of body mass index [published online ahead of print August 16, 2012]. JPEN J Parenter Enteral Nutr. doi:10.1177/0148607112457423.
- Frankenfield DC, Coleman A. Recovery to resting metabolic state after walking. J Am Diet Assoc. 2009;109(11):1914-1916.
- Frankenfield DC, Rowe WA, Smith JS, Cooney RN. Validation of several established equations for resting metabolic rate in obese and nonobese people. J Am Diet Assoc. 2003;103(9):1152-1159.
- Frankenfield DC, Schubert A, Alam S, Cooney RN. Analysis of Estimation Methods for Resting Metabolic Rate in Critically Ill Adults. JPEN J Parenter Enteral Nutr. 2009;33:27-36.
- Frankenfield DC, Smith JS, Cooney RN, Blosser SA. Relative association of fever and injury with hypermetabolism in critically ill patients. Injury. 1997;28(9-10):617-621.
- Frankenfield DC, Wiles CE, Bagley SM, Siegel JH. Relationships between resting and total energy expenditure in trauma and septic patients. Crit Care Med. 1994;22(11):1796-1804.
- Gariballa S, Forster S. Energy expenditure of acutely ill hospitalised patients. Nutr J. 2006;5:9.
- Govan MI, Poehlman ET. Endurance training does not enhance total energy expenditure in healthy elderly persons. Am J Physiol. 1992;263(26):E950-E957.
- Heidegger CP, Graf S, Thibault R, Darmon P, Berger MM, Pichard C. Supplemental parenteral nutrition (SPN) in intensive care unit (ICU) patients for optimal energy coverage: improved clinical outcome. Clin Nutr. 2011;6(suppl):2-3.
- Heymsfield SB, Hill JO, Evert M, Casper K, Digirolamo M. Energy expenditure during continuous intragastric infusion of fuel. Am J Clin Nutr. 1987;45(3):526-533.
- Hoffer LJ. Protein and energy provision in critical illness. Am J Clin Nutr. 2003;78(5):906-911.
- Ireton-Jones CS, Turner WW, Liepa GU, Baxter CR. Equations for the estimation of energy expenditure in patients with burns with special reference to ventilator status. J Burn Care Rehabil. 1992;13(3):330-333.
- Kosmiski L. Energy expenditure in HIV infection. Am J Clin Nutr 2011;94(suppl):1677S–82S.
- Livingston EH, Kohlstadt I. Simplified resting metabolic rate-predicting formulas for normal-sized and obese individuals. Obes Res. 2005;13(7):1255-1262.
- Marik PE, Hooper MH. Normocaloric versus hypocaloric feeding on the outcomes of ICU patients: a systematic review and meta-analysisIntensive Care Med. 2016 Mar;42(3):316-23.
- Mifflin MD, St Jeor ST, Hill LA, Scott BJ, Daugherty SA, Koh YO. A new predictive equation for resting energy expenditure in healthy individuals. Am J Clin Nutr. 1990;51(2):241-247.
- Muller MJ, Bosy-Westphal A, Kutzner D, Heller M. Metabolically active components of fat-free mass and resting energy expenditure in humans: recent lessons from imaging technologies. Obes Rev. 2002;3(2):113-122.
- Singer P, Anbar R, Cohen J, Shapiro H, Shalita-Chesner M, Lev S, Grozovski E, Theilla M, Frishman S, Madar Z. The tight calorie control study (TICACOS): a prospective, randomized, controlled pilot study of nutritional support in critically ill patients. Intensive Care Med. 2011;37(4):601-609.
- U.S. Department of Health and Human Services and U.S. Department of Agriculture. 2015–2020 Dietary Guidelines for Americans. 8th Edition. July 2018.
- Van der Kuip M, de Meer K, Westerterp KA, Gemke RJ. Physical activity as a determinant of total energy expenditure in critically ill children. Clin Nutr. 2007;26(6):744-751.
- olp ACP, de Oliveira E, Alves RDM, Esteves EA, Bressan J. Energy expenditure: components and evaluation methods. Nutr Hosp. 2011;26(3):430-440.
- Wang Z, Ying Z, Bosy-Westphal A, Zhang J, Heller M, Later W, Heymsfield SB, Müller MJ. Evaluation of specific metabolic rates of major organs and tissues: comparison between nonobese and obese women. Obesity (Silver Spring). 2012 Jan;20(1):95-100. doi: 10.1038/oby.2011.256. Epub 2011 Aug 11.
- Weijs PJM, Kruizenga HM, van Dijk AE, van der Meij BS, Langius JAE, Knol DL, Strack van Schijndel RJM, van Bokhorst-de van der Schueren MAE. Validation of predictive equations for resting energy expenditure in adult outpatients and inpatients. Clin Nutr. 2008;27(1):150-157.
- Weissman C, Kemper M, Damask MC, Askanazi J, Hyman AI, Kinney JM. Effect of routine intensive care interactions on metabolic rate. Chest. 1984;86(6):815-818.
